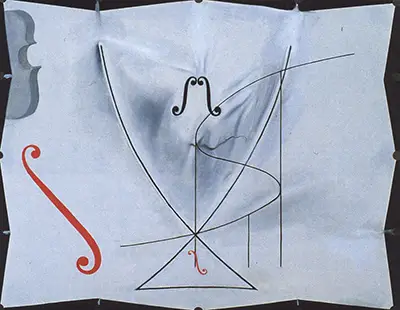
Thom, a highly respected French mathematician and Fields Medal winner proposed a catastrophe theory that, according to Dali, was “the most beautiful aesthetic theory in the world”.
Thom’s theory suggests that in four-dimensional space there are seven equilibrium surfaces: swallowtail, butterfly, fold, cusp, elliptic umbilic, parabolic umbilic and hyperbolic umbilic, and Dali, perhaps because of his admiration for Thom’s work, undertook the not-inconsiderable challenge of successfully representing this relatively intangible four-dimensional theory on a two-dimensional canvas.
The shape of the swallow’s tail at the bottom of the painting for example, is copied from the mathematician’s graph of the same name, and the f-holes of the cello in the painting describe the integral symbol in calculus.
Similarly, the S curve represents Thom’s second catastrophe graph. Given the often-considerable differences in thinking between artist and mathematician (although the lines purportedly blur the more theoretical the mathematics becomes) the relationship between Dali and Thom is remarkable.
The mathematician’s acceptance at the only meeting between the two men – whether tongue in cheek or genuine – that a railway station in France was indeed the centre of the universe implies a human connection at some level, and indeed Dali was influenced by this statement in a separate painting: Topological Abduction of Europe – Homage to Rene Thom.
Interestingly the theory itself, initially met with scepticism, became increasingly popular after its initial proposition and Dali’s embrace and remarkable representation of the theory on canvas makes this a striking last painting of this extraordinary artist’s life.